Examples, solutions, videos, and worksheets to help Grade 6 students learn how to evaluate numbers with negative exponents. The number, also called the base, can be a positive or negative whole number, a positive or negative fraction, a positive or negative decimal.
There are four sets of negative exponent worksheets:
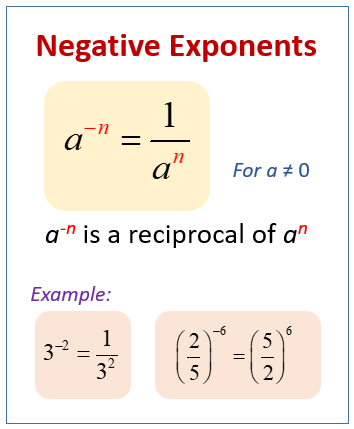
When a number is raised to a negative exponent, it means that we take the reciprocal of that number raised to the positive exponent.
Here are some examples:
2 -3 :
This means taking the reciprocal of 2 3 , which is 1/2 3 . Therefore, 2 -3 = 1/(2 3 ) = 1/8 = 0.125.
(1/3) -4 :
This means taking the reciprocal of (1/3) 4 , which is 3 4 . Therefore, (1/3) -4 = 3 4 = 81.
(2/3) -2 :
This means taking the reciprocal of (2/3) 2 , which is (3/2) 2 . Therefore, (2/3) -2 = (3/2) 2 = 9/4.
0.2 -2 :
This means taking the reciprocal of 0.2 2 , which is 1/0.2 2 . Therefore, 0.2 -2 = 1/(0.2 2 ) = 1/0.04 = 25.
We can also change the decimals to fractions before evaluating.
0.2 -2 = (2/10) -2 = (10/2) 2 = 100/4 = 25
So, when dealing with negative exponents, you can think of it as taking the reciprocal of the positive exponent.

Click on the following worksheet to get a printable pdf document.
Scroll down the page for more Negative Exponents Worksheets.

Printable
(Answers on the second page.)
Negative Exponents Worksheet #1 (Positive Whole Number Bases)
Negative Exponents Worksheet #2 (Negative Whole Number Bases)
Negative Exponents Worksheet #3 (Positive or Negative Fractional Bases)
Negative Exponents Worksheet #4 (Positive or Negative Decimal Bases)
 Mathway Calculator Widget" width="320" height="267 loading=" />
Mathway Calculator Widget" width="320" height="267 loading=" />
Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.